-
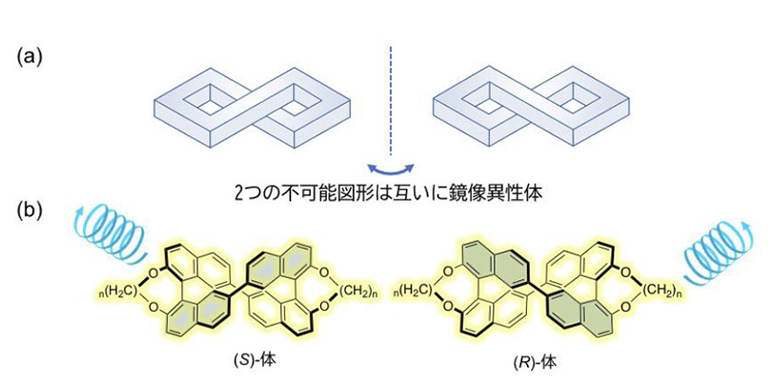
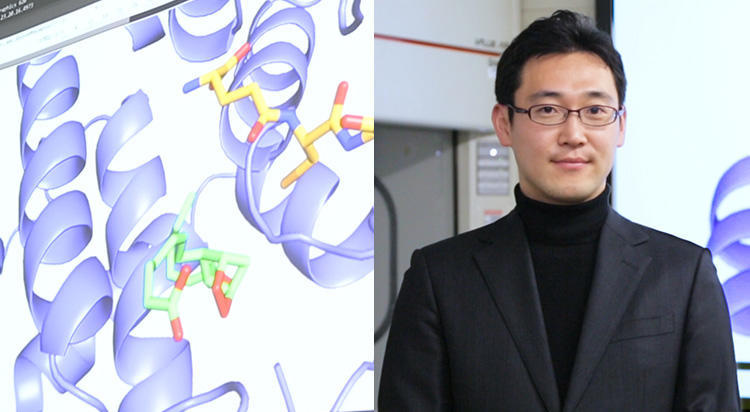
Successful development of luminophores with strong CPL due to organic molecules with trompe l'oeil-like geometry
High expectations for polarized luminescent-type 3D display materials -
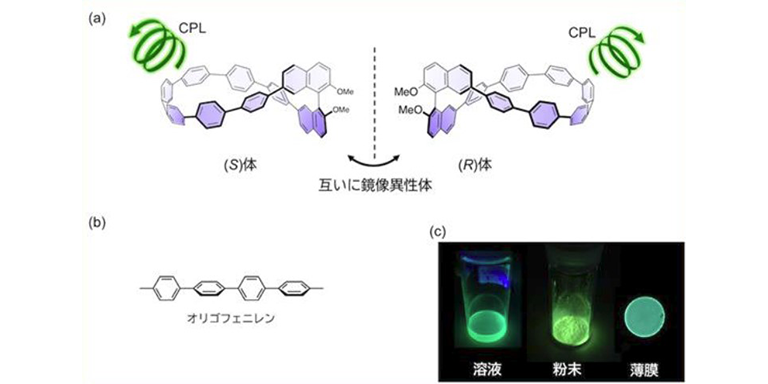
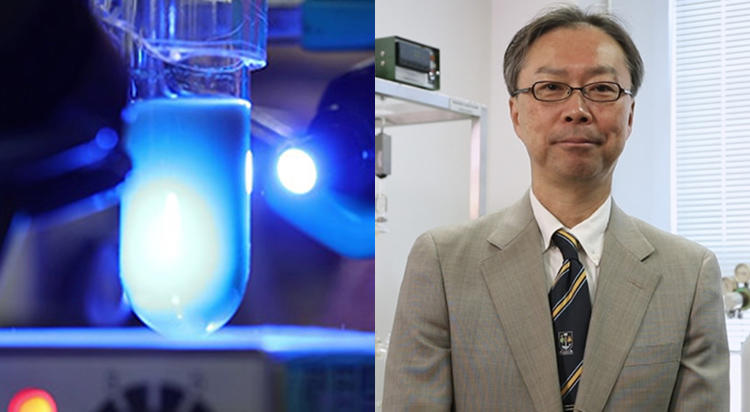
CPL dye developed with spiral-shaped twisted oligophenylene
Promising for applications such as circularly polarized organic LED and bioimaging materials -

Method developed for replicating ocean air currents and waves inside experimental water tanks
Hopefully will help improve reliability of typhoon and other climate forecasts
-
Kengo Oka, lecturer in the Applied Chemistry Department, wins FY2020 advancement award from Ceramic Society of Japan
2021.03.31
-
Two students in the Informatics Department each win student encouragement award at 83rd IPSJ national convention
2021.03.22
-
Mizuki Koike, graduate student in Electronic Engineering, wins ITE outstanding student presentation award
2021.03.22
-
Takuto Mibu, doctoral candidate in Science, wins poster award at 1st Asian Conference on Molecular Magnetism
2021.03.15
-
Department of Applied Chemistry and Chemistry Course hold joint company briefing session
2021.03.02
-
Successful development of luminophores with strong CPL due to organic molecules with tromp...
2021.02.25
-
JST to support innovative research by Dr. Ippei Danshita to discover novel quantum many-bo...
2021.02.04
-
CPL dye developed with spiral-shaped twisted oligophenylene
Promising for application...2020.12.10
-
Hal Suzuki, lecturer in the Chemistry Course, wins FY2020 Research Encouragement Award fro...
2020.12.01
-
Joint research by 3 graduate students majoring in Electronic Engineering appear in APS Phy...
2020.11.30
-
Bio-Coke Research Institute holds seminar for kids on renewable energy
2020.12.10
-
Kindai University's Mathematics Contest marks 22nd anniversary
Special online version uses questions selected from past events2020.10.21
-
13th joint information session on universities for learning about outer space held online
2020.07.31
-
2nd Faculty of Sci.& Eng. cross-disciplinary exchange meeting
2015.05.06
-
Lectures by Prof. Sebbar of Bordeaux University
2015.04.16
-
Department of Applied Chemistry and Chemistry Course hold joint company briefing session
2021.03.02
-
TV appearances by Kindai University faculty members in January and February 2021
2021.01.13
-
Mathematical Seminar of Kindai University
2019.07.05
-
Dr. Nagatoshi Koumura for his lecture on "Discovery of molecular rotors that can be driven by photo-energy and Elucidation of the mechanisms".
2017.06.16
-
Students of Soft Matter Physics Laboratory attended the Fifth Soft Matter Workshop
2015.12.22
-
Kengo Oka, lecturer in the Applied Chemistry Department, wins FY2020 advancement award from Ceramic Society of Japan
2021.03.31
-
Two students in the Informatics Department each win student encouragement award at 83rd IPSJ national convention
2021.03.22
-
Mizuki Koike, graduate student in Electronic Engineering, wins ITE outstanding student presentation award
2021.03.22
-
Takuto Mibu, doctoral candidate in Science, wins poster award at 1st Asian Conference on Molecular Magnetism
2021.03.15
-
Akihiro Ito, fourth-year student in Department of Electric and Electronic Engineering, wins award from IEEJ Magnetics Society
2021.02.20