Academic Staff and Fellows

SUZUKI Takao: Associate Professor Ph.D (Science) (Kobe University); Department/Science　 Graduate school/Science

My research field is the theory of special functions which are defined by differential or difference equations in complex domains. These special functions are related to various fields of pure mathematics and applied mathematics. Therefore they are very interesting research object.

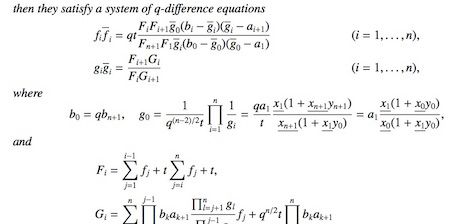
Discrete Painlevé system of type A

Research Area	Special functions and Integrable systems
Research Interests	Painleve equations Hypergeometric functions Infinite dimensional integrable systems
Selected Publications	(1) A Lax Formulation of a Generalized q-Garnier System, Takao Suzuki, Math. Phys. Anal. Geom. 24(4) (2021). (2) Generalized q-Painlevé system of type (A_2n+1+A₁+A₁)⁽¹⁾arising from cluster algebra, Naoto Okubo and Takao Suzuki, Int. Math. Res. Not. 2022(9) 6561-6607 (2022). (3) An affine Weyl group action on the basic hypergeometric series arising from the q-Garnier system, Taiki Idomoto and Takao Suzuki, Lett. Math.Phys. 112(6) (2022).
Affiliated Academic Societies	The Mathematical Society of Japan
Education (Undergraduate Course)	Kobe University
Education (Master's/Doctral Course)	Kobe University
Title of Thesis, Institute, Date	Affine Weyl group symmetry of the Garnier system, Kobe University, March 2004

Special Functions Laboratory

E-mail	suzuki（at）math.kindai.ac.jp Note that this e-mail address has replaced the "@" with "(at)" to prevent spam. When e-mailing, replace the "(at)" with "@".